2020年度 センター試験 数学II 第2問
(1)
より,
の方程式は,
より,
の方程式は,
係数を比較して,
これを解いて,
の方程式は,
→ア=2, イ=2, ウ=1, エ=2, オ=4, カ=2, キ=4, ク=1, ケ=0, コ=2, サ=2, シ=1
(2)
と
を解くと,

と
の交点は,
と
を解いて,
→ス=a, セ=3, ソ=3
(3)
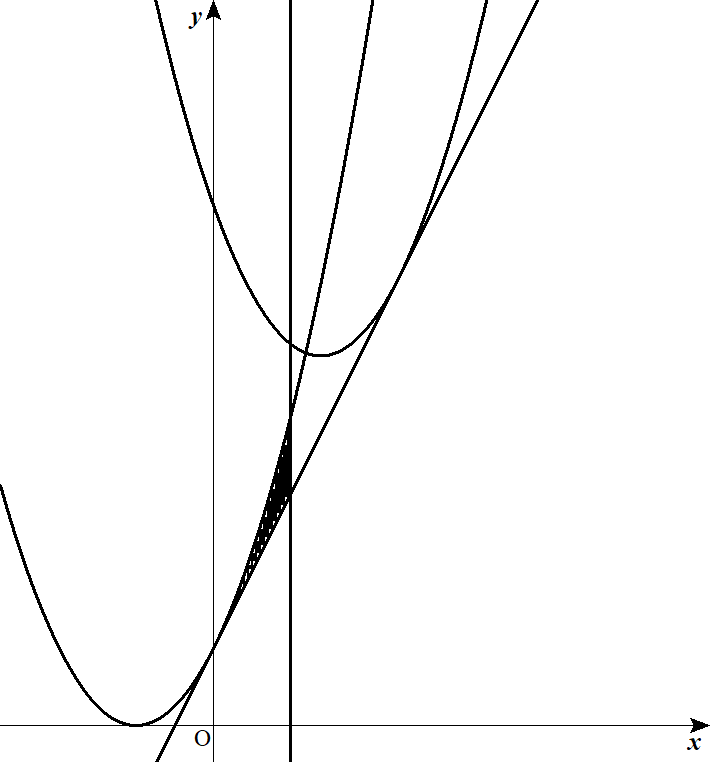
の交点は
より,
のとき,
のとき,
→タ=1, チ=1, ツ=3, テ=2, ト=4, ナ=2, ニ=1, ヌ=3
(4)
は
で最大値
をとる
→ネ=2, ノ=3, ハ=2, ヒフ=27