2020年度 センター試験 数学I 第1問
〔1〕
(1)
の傾きは,
だから,
→アイ=-2, ウ=4
(2)
を代入して,
の場合,
の場合,
また, のとき,
→エ=0, オ=4, カキ=-2, ク=5, ケ=3, コ=6, サシ=13
(3)
より,
このとき,
のとき,
→スセ=-1, ソ=2, タチツ=-14, テト=13
〔2〕
(1)
→ナ=2, ニ=5
(2)
また,
→ヌネ=12, ノ=4
(3)
→ハ=3
2020年度 センター試験 数学II・数学B 第4問
(1)
→ア=3, イ=6, ウ=4, エ=3, オカ=36
(2)
より,
より,
これらを解いて,
→キク=-2, ケ=3, コ=1, サ=2, シ=6
(3)
より,
四角形OABCは平行四辺形ではないが台形である
また, より,
四角形OABCの面積は,
→ス=2, セ=2, ソタ=-4, チ=3, ツテ=30
(4)
とおくと,
これらを解いて,
このとき,
より,

三角形ABCを底面とする四面体DABCの高さは,
四面体DABCの体積は,
→ト=1, ナ=2, ニ=2, ヌ=1, ネ=2, ノ=2, ハヒ=60, フ=3, ヘ=4, ホ=3
2020年度 センター試験 数学II・数学B 第3問
(1)
→ア = 6
(2)
の両辺を
で割ると,
→イ=0, ウ=1, エ=1, オ=2, カ=3, キ=1
を
以上の自然数とするとき,
これはのときも成り立つ
→ク=2, ケ=1, コ=1, サ=1, シ=6, ス=1, セ=2, ソ=2, タ=3, チ=1
(3)
(2)より,
→ツ=3, テ=1, ト=4, ナ=1, ニ=2, ヌ=3
(4)
のとき,
を
で割った余りは,
を
で割った余りに等しい
を
で割った余りは,
よりそれぞれ
また, の初項から第
項までの和を
で割った余りは
より,
を
で割った余りに等しく,
→ネ=1, ノ=0, ハ=0, ヒ=1
2020年度 センター試験 数学II・数学B 第2問
(1)
より,
の方程式は,
より,
の方程式は,
係数を比較して,
これを解いて,
の方程式は,
→ア=2, イ=2, ウ=1, エ=2, オ=4, カ=2, キ=4, ク=1, ケ=0, コ=2, サ=2, シ=1
(2)
と
を解くと,

と
の交点は,
と
を解いて,
→ス=a, セ=3, ソ=3
(3)
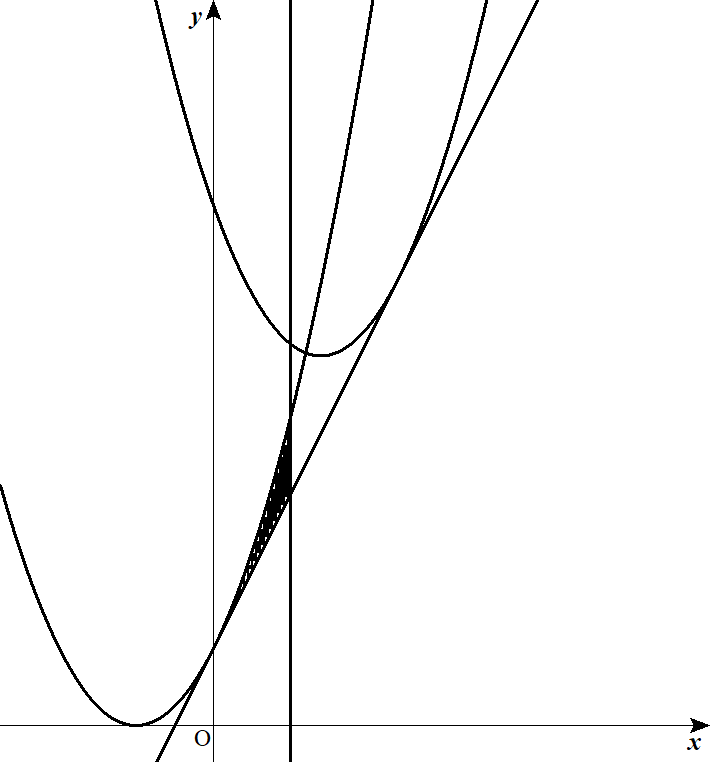
の交点は
より,
のとき,
のとき,
→タ=1, チ=1, ツ=3, テ=2, ト=4, ナ=2, ニ=1, ヌ=3
(4)
は
で最大値
をとる
→ネ=2, ノ=3, ハ=2, ヒフ=27
2020年度 センター試験 数学II・数学B 第1問
〔1〕
(1)
加法定理より,
→ア=3, イ=2, ウ=3, エ=3, オ=2, カ=3, キ=5, ク=3
(2)
解と係数の関係より,
このとき,
のとき,
このとき,
→ケコ=12, サ=4, シ=5, ス=3, セ=5, ソ=3
〔2〕
(1)
のとき,
→タチ=11, ツテ=13, トナニ=-36
(2)

上図より, のとり得る最大の整数の値は
のとき,
これを満たす最大の整数の値は
→ヌ=2, ネノ=10, ハ=3, ヒフ=-4, ヘ=7, ホ=5
2020年度 センター試験 数学I・数学A 第5問

チェバの定理より,
メネラウスの定理より,
→ア=1, イ=1, ウ=8, エ=2, オ=7
→カ=9, キク=56
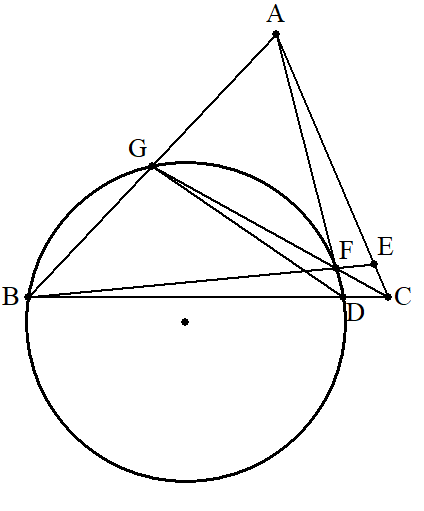
方べきの定理より,
のとき,
→ケコ=12, サシ=72
また, より,
方べきの定理の逆より,
4点G, B, C, Eは同一円上にある
→ス=2